Understanding The Essence Of Functions: Domain And Range In Mathematics

Understanding the Essence of Functions: Domain and Range in Mathematics

Related Articles: Understanding the Essence of Functions: Domain and Range in Mathematics

Introduction

In this auspicious occasion, we are delighted to delve into the intriguing topic related to Understanding the Essence of Functions: Domain and Range in Mathematics. Let’s weave interesting information and offer fresh perspectives to the readers.

Understanding the Essence of Functions: Domain and Range in Mathematics

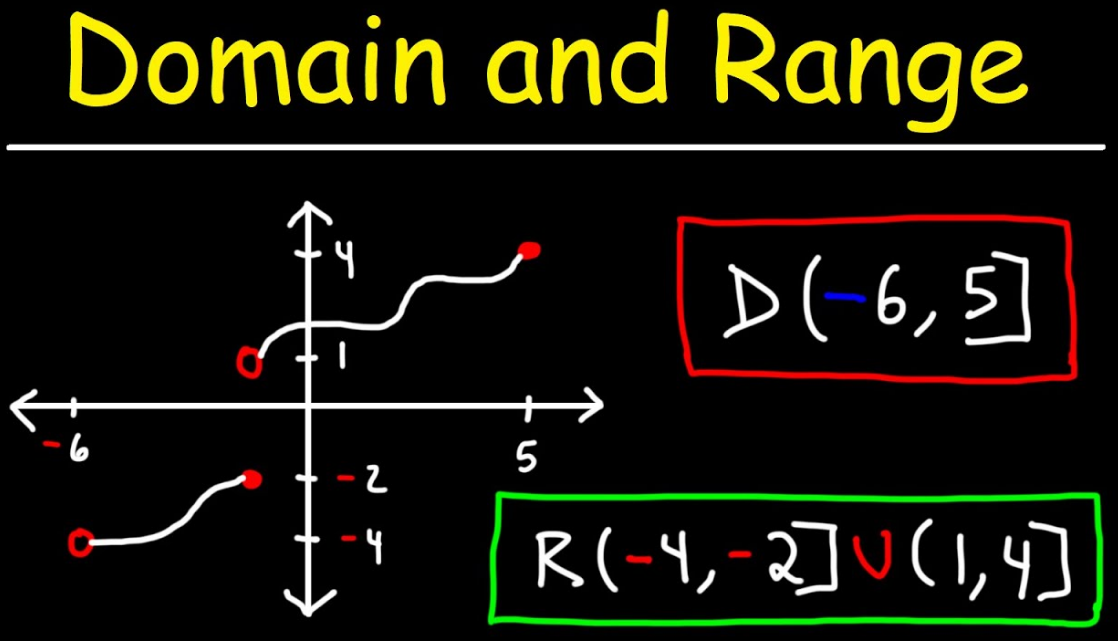
The study of functions in mathematics is fundamental to understanding relationships between variables. A function, in essence, describes a specific correspondence between elements of two sets. To fully grasp this correspondence, we must consider two key concepts: domain and range.

Domain: The Set of Inputs

The domain of a function represents the complete set of all possible input values for which the function is defined. These input values are typically represented by the independent variable, often denoted by ‘x’. Think of the domain as the "allowed" values that can be plugged into the function.

Range: The Set of Outputs

The range of a function, on the other hand, encompasses all possible output values that the function can produce. These output values are typically represented by the dependent variable, often denoted by ‘y’. The range represents the "results" obtained when applying the function to the values within the domain.

Visualizing Domain and Range

Imagine a function as a machine. You feed it an input (from the domain), and it processes it to generate an output (from the range).

• Domain: The set of all possible ingredients you can put into the machine.
• Range: The set of all possible dishes the machine can produce.

Illustrative Examples

Let’s consider some examples to solidify the concepts of domain and range:

Example 1: Linear Function

Consider the linear function: f(x) = 2x + 1

• Domain: All real numbers. This is because we can plug in any real number for ‘x’ and obtain a valid output.
• Range: All real numbers. As ‘x’ varies over the entire real number line, the output ‘y’ also varies over the entire real number line.

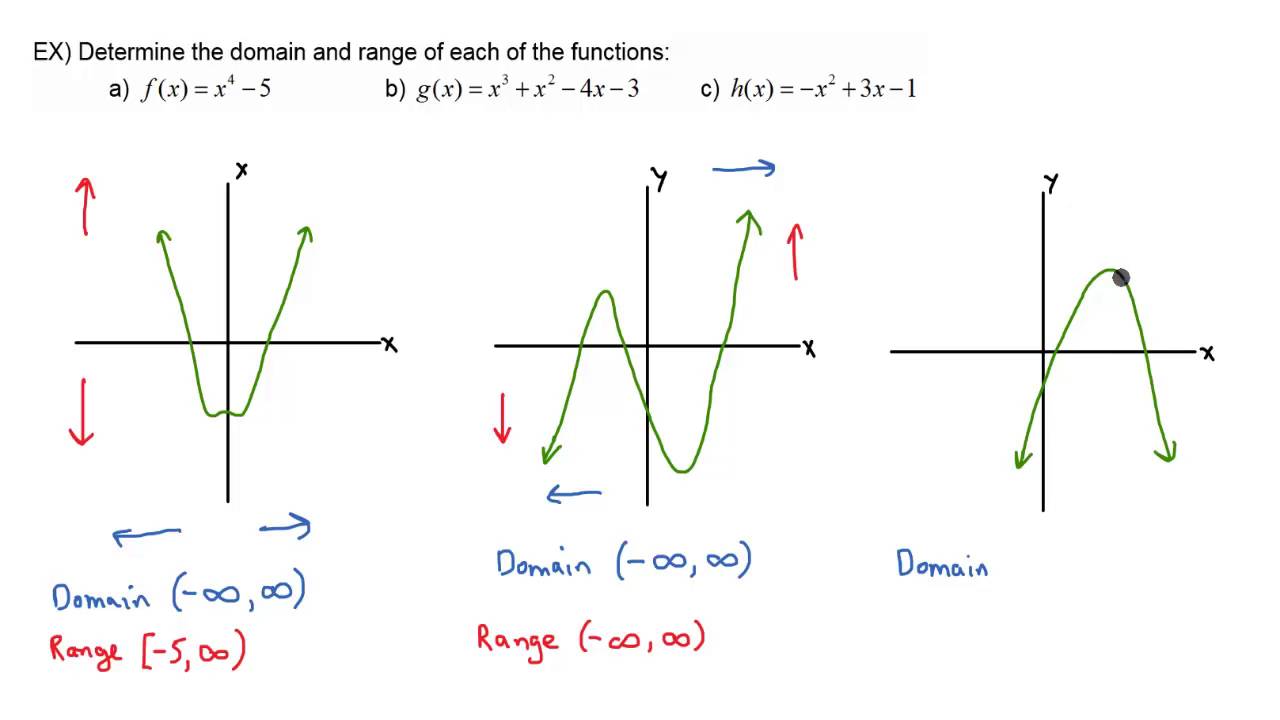
Example 2: Quadratic Function

Consider the quadratic function: f(x) = x²

• Domain: All real numbers. We can square any real number.
• Range: All non-negative real numbers. The square of any real number is always non-negative.

Example 3: Square Root Function

Consider the square root function: f(x) = √x

• Domain: All non-negative real numbers. We cannot take the square root of a negative number.
• Range: All non-negative real numbers. The square root of a non-negative number is always non-negative.

Determining Domain and Range

To determine the domain and range of a function, we can use various techniques:

1. Algebraic Analysis:

• Identify any restrictions: Look for values that would make the function undefined, such as division by zero, taking the square root of a negative number, or taking the logarithm of a non-positive number.
• Exclude these restrictions: The domain will be all real numbers except for the excluded values.
• Analyze the function’s behavior: Consider the function’s behavior as the input varies. This can help determine the possible output values and thus the range.

2. Graphical Analysis:

• Plot the function: Graphing the function provides a visual representation of its behavior.
• Identify the x-values: The x-values where the graph exists represent the domain.
• Identify the y-values: The y-values covered by the graph represent the range.

3. Using Set Notation:

• Interval notation: This notation uses parentheses and brackets to represent ranges of values. For example, (2, 5) represents all values between 2 and 5, excluding 2 and 5.
• Set-builder notation: This notation describes the set using a rule. For example, x represents the set of all numbers greater than 0.

The Importance of Domain and Range

Understanding domain and range is crucial for several reasons:

• Function definition: Domain and range define the function’s complete behavior, outlining the permissible inputs and the resulting outputs.
• Function analysis: Domain and range help in analyzing the function’s behavior, identifying its limits, and determining its properties.
• Problem-solving: Domain and range play a vital role in solving real-world problems that can be modeled using functions. For example, in physics, the domain and range of a function describing the motion of an object can provide insights into its trajectory and speed.

FAQs about Domain and Range

1. Can the domain and range of a function be the same?

Yes, they can be the same. For example, the function f(x) = x has a domain and range of all real numbers.

2. Is the domain always a set of real numbers?

No, the domain can be a set of any type of elements, including integers, complex numbers, or even sets themselves.

3. Can the range be a single value?

Yes, the range can be a single value. For example, the function f(x) = 5 has a range of 5.

4. How do I find the domain of a function that involves fractions?

The denominator of a fraction cannot be zero. Therefore, you must exclude any values of the input variable that make the denominator zero.

5. How do I find the range of a function that involves a square root?

The square root of a number cannot be negative. Therefore, the range of a function involving a square root will be all non-negative numbers.

Tips for Understanding Domain and Range

• Start with simple functions: Begin by understanding the domain and range of basic functions like linear, quadratic, and square root functions.
• Visualize the function: Graphing the function can help visualize the domain and range.
• Identify restrictions: Look for any restrictions on the input values that would make the function undefined.
• Use set notation: Practice using interval and set-builder notation to represent domain and range.
• Apply to real-world problems: Try applying the concepts of domain and range to real-world scenarios to understand their practical implications.

Conclusion

Understanding the concepts of domain and range is fundamental to grasping the behavior of functions. They define the permissible inputs and the resulting outputs, providing a complete picture of the function’s behavior. By mastering these concepts, we can effectively analyze functions, solve problems, and gain deeper insights into mathematical relationships. Remember to always consider the restrictions on the input values and analyze the function’s behavior to accurately determine the domain and range. These concepts are essential tools in the study of mathematics and have wide-ranging applications in various fields.

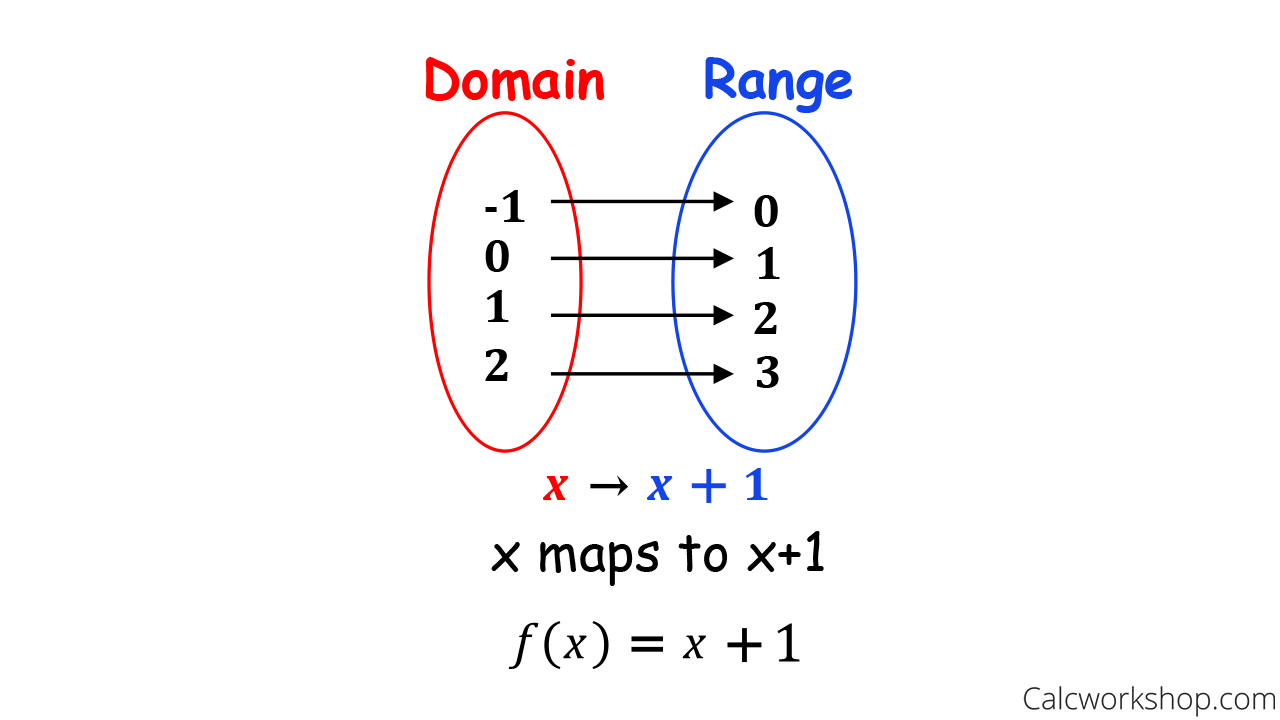
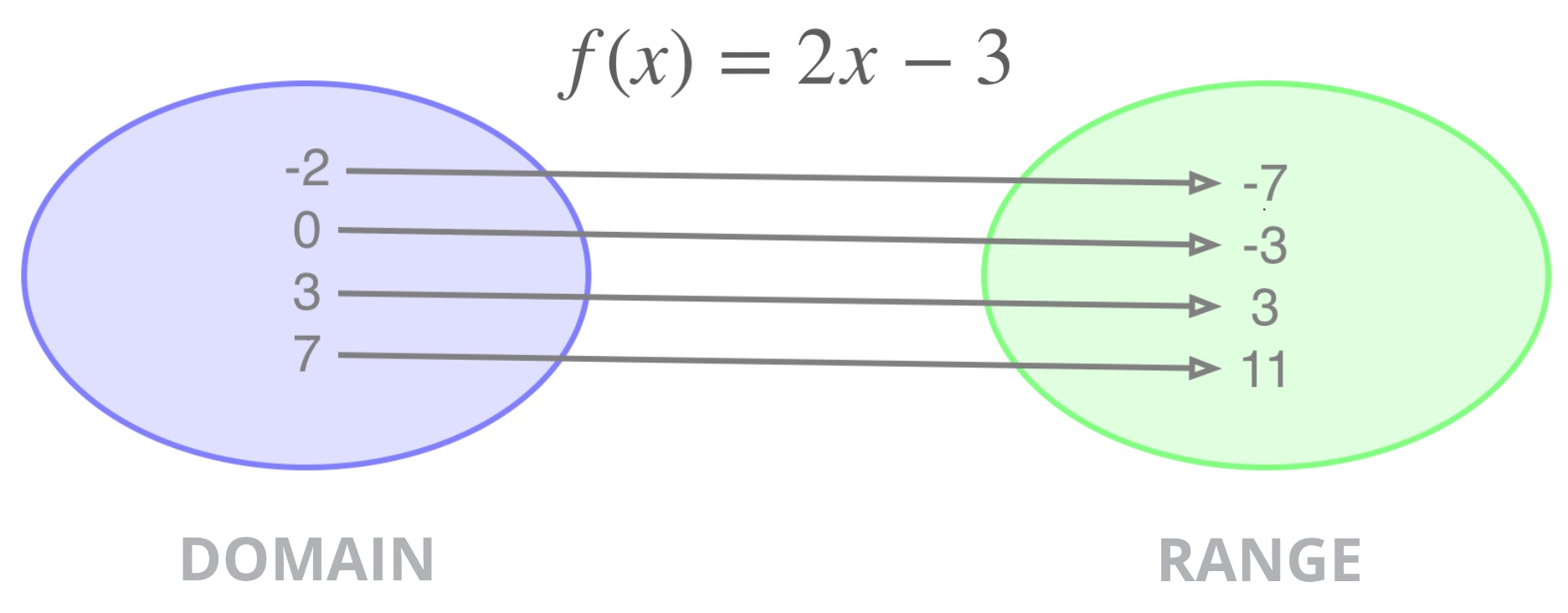
Closure

Thus, we hope this article has provided valuable insights into Understanding the Essence of Functions: Domain and Range in Mathematics. We appreciate your attention to our article. See you in our next article!